Simplify the Following Expressions and Write the Results in the Polar Form R Eiãžâ¸ .

A complex number can be called a hybrid of real and imaginary numbers, with the real number or constituent being any fraction, rational or irrational integer and its imaginary part being represented as a real number in multiplication with the imaginary unit iota, depicted i. Thus, a complex number shows a real number and an imaginary number combined by either of these two arithmetic operations, addition, and subtraction.

Real and Imaginary numbers

Such numbers which include both rational numbers and their irrational counterparts are called real numbers. They are based on the concept of a number line, with zero being the origin and all the numbers to its right, positive, and those in the left of the origin, negative.

Hey! Looking for some great resources suitable for young ones? You've come to the right place. Check out ourself-paced courses designed for students of grades I-XII.

Start with topics likePython, HTML, ML, and learn to make some games and apps all with the help of our expertly designed content! So students worry no more, becauseGeeksforGeeks School is now here!

Numbers can also be depicted as the square root of a negative number in mathematics. For example, is an imaginary number, since it depicts the number 100, which is a perfect square as a negative number under a square root. Such numbers are not tangible, but still kind of real in the sense that they are used in mathematics. In other words, imaginary numbers are numbers that are the opposite of real numbers. They are not based on the concept of the number line, and as a result, cannot be depicted or plotted on one. Another way of defining an imaginary number could be such a number that yields a negative result when multiplied with itself, i.e., squared.

Standard Form of a Complex Number

A complex number, in its standard form, is expressed as a + ib, where a and b both are real numbers, but b being in multiplication with the imaginary variable i, represents the imaginary part of the whole complex number, which can be denoted by 'z'. Hence, a complex number is usually written in the form z = a + ib, where a depicts the real part and ib or bi would be the imaginary constituent. For that matter, 0 + bi would also be regarded as a complex number with the real part being non-existent and bi depicting its imaginary counterpart. Examples are,

• 5 + 2i is a complex number, where 5 is the real part and 2i depicts the imaginary part.
• e² + 12i  is a complex number, where e2 is the real part and 12i is the imaginary part.
• √22 – 162i is a complex number, where √22 is the real part and 162i is the imaginary part.

Polar Form of a Complex Number

The standard form of a complex number can also be called its rectangular form. The polar form is just another way of expressing a complex number, with the help of its modulus and argument. The polar form is represented using the polar coordinates of the real and imaginary components of the given complex numeral.

The equation of polar form of z = x + iy is z = r(cosθ + i sinθ).

Here, z = r(cosθ + i sinθ), where r = |z| =

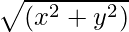
x = r cosθ, y = r sinθ.

How to use De Moivre's theorem to simplify (1 – i)¹⁰?

Solution:

De Moivre's Theorem

This theorem holds utmost importance in the universe of complex numbers as it helps connect the field of trigonometry to the intricacies of complex numerals. It also helps obtain relationships between various trigonometric functions of different angles. It is so-called because this theorem was propounded by one of the most notable mathematicians in history, De Moivre, who contributed a lot to the fields of probability, algebra, etc. The theorem is also referred to as De Moivre's Formula or De Moivre's Identity.

Formula

For a number x, such that x ∈ R, or for all real values of x,

(cos x + i sin x)ⁿ = cos nx  + i sin nx

Or (e^iθ)ⁿ= e^inθ

Here, n is a rational number and i, called iota is the imaginary part.

r =, θ = π/4

The polar form of  (1 – i) =

According to De Moivre's Theorem: (cosθ + sinθ)ⁿ = cos(nθ) + isin(nθ).

Thus, (1 – i)¹⁰ =

=

= 32 [0 + i(-1)]

= 32 (-i)

Hence, (1 – i)¹⁰ = 0 – 32i.

Similar Problems

Question 1: Simplify (1 + i)⁵ using De Moivre's Theorem.

Solution:

Here, r =, θ = π/4

The polar form of (1+i) =

According to De Moivre's Theorem: (cosθ + sinθ)ⁿ = cos(nθ) + i sin(nθ).

Thus, (1+i)⁵ =

=

= -4 – 4i

Hence, (1 + i)⁵ = -4 – 4i.

Question 2: Simplify (2 + 2i)⁶ using De Moivre's Theorem.

Solution:

Here, r =, θ = π/4

The polar form of  (2+2i) =

According to De Moivre's Theorem: (cosθ + sinθ)ⁿ = cos(nθ) + isin(nθ).

Thus, (2 + 2i)⁶ =

=

= 512 (-i)

Hence, (2 + 2i)⁶ = −512i.

Question 3: Simplify (1 + i)¹⁸ using De Moivre's Theorem.

Solution:

Here, r =, θ = π/4

The polar form of  (1+i) =

According to De Moivre's Theorem: (cosθ + sinθ)ⁿ = cos(nθ) + isin(nθ).

Thus, (1+i)¹⁸ =

=

= 512i

Hence, (1 + i)¹⁸ = 512i.

Question 4: Simplify (-√3 + 3i)³¹ using De Moivre's Theorem.

Solution:

Here, r =, θ = 2π/3

The polar form of  (-√3 + 3i) =

According to De Moivre's Theorem: (cosθ + sinθ)ⁿ = cos(nθ) + isin(nθ).

Thus, (-√3 + 3i)³¹=

=

Hence, (-√3 + 3i)31 =.

Simplify the Following Expressions and Write the Results in the Polar Form R Eiãžâ¸ .

Source: https://www.geeksforgeeks.org/how-to-use-de-moivres-theorem-to-simplify-1-i10/

Share this post